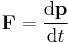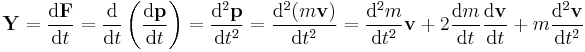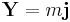Yank (physics)
In physics, yank is the derivative of force with respect to time[1]. Expressed as an equation, yank Y is:
where F is force and  is the derivative with respect to time
is the derivative with respect to time  .
.
The term yank is not universally recognized but is commonly used. The units of yank are force per time, or equivalently, mass times length per time cubed; in the SI unit system this is kilogram metres per second cubed (kg·m/s3), or Newtons per second (N/s).
Relation to other physical quantities
Newton's second law of motion says that:
where p is momentum, so if we combine the above two equations:
where 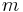 is mass and v is velocity. If the mass isn't changing over time (i.e. it's constant), then:
is mass and v is velocity. If the mass isn't changing over time (i.e. it's constant), then:
which can also be written as:
where j is jerk.
References
- ^ Gragert, Stephanie (November 1998). "What is the term used for the third derivative of position?". Usenet Physics and Relativity FAQ. Math Dept., University of California, Riverside. http://math.ucr.edu/home/baez/physics/General/jerk.html. Retrieved 2008-03-12.